Mark the angles first.
Consider the diagram below,
Given that angle A and K measure 124 degree, and 96 degree.
Since line 'c' and 'd' are parallel, then being the corresponding angles,
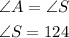
Angles S and T together constitute a straight line, so they must be supplementary,

Now, since lines 'f' and 'g' are parallel, so the angles W and T must also be equal, being the corresponding angles,
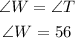
Thus, the measure of angle W, i.e. 'x' is 56 degrees.