Question

Towards the end of the day, you (m= 62 kg) and one of your friends (m = 71 kg) decide toride one of the smaller slides. The slide is 6.7 m tall and you start at the top of the slide fromrest. How fast are you traveling when you are half way down the slide if friction is negligible?Water park rules state that riders must wait until the rider in front of them has finished sliding and has cleared the landing area before they can slide; however, your friend doesn't listen and decides to go shortly after you go. You both collide and stick together at the halfway point. If Your friend is moving at a velocity of 8.5 m/s when the collision occurs, how fast are you bothmoving after the collision? You both remain together after the collision and continue to slide to the bottom from the half way point. How fast are you both moving at the bottom of the slide?

Answer 1

Using conservation of energy:

`\begin{gathered} E1=E2 \\ mgh1=(1)/(2)mv2^2+mgh2 \end{gathered}`

Solve for v2:

`\begin{gathered} 2gh1=v2^2+2gh2 \\ v2=√(2g(h1-h2)) \\ v2=√(2(9.8)(6.7-6.7/2)) \\ v2\approx8.1m/s \end{gathered}`

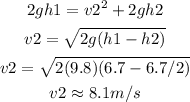
When you are half way down, your velocity is approximately 8.1 m/s

Using conservation of momentum:

`m1va+m2vb=v(m1+m2)`

Where:

m1 = Your mass = 62kg

m2 = your friend's mass = 71kg

va = Your velocity = 8.1m/s

vb = Your friend's velocity = 8.5m/s

v = Velocity after the collision

so:

`\begin{gathered} v=(m1va+m2vb)/(m1+m2) \\ v=(62\cdot8.1+71\cdot8.5)/(62+71) \\ v=(502.2+603.5)/(133) \\ so: \\ v=(1105.7)/(133) \\ v\approx8.3m/s \end{gathered}`

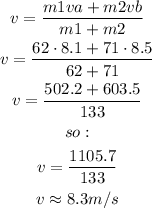
After the collision you and your friend are moving at 8.3 m/s

Finally, using conservation of energy again:

`\begin{gathered} E2=E3 \\ (1)/(2)(m1+m2)v^2+(m1+m2)gh2=(1)/(2)(m1+m2)vf^2 \end{gathered}`

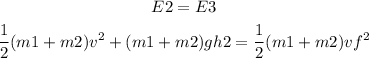
Where:

vf = velocity at the bottom

so:

`v^2+2gh2=vf^2`

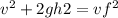
Solve for vf:

`\begin{gathered} vf=√(v^2+2gh2) \\ vf=√(8.3^2+2(9.8)(3.35)) \\ vf=√(68.89+65.66) \\ vf=√(134.55) \\ vf\approx11.6m/s \end{gathered}`

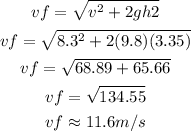
At the bottom of the slide the velocity of you and your friend together is 11.6 m/s