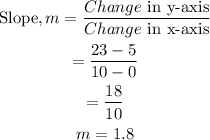Part A
The equation that gives the total cost, y to travel x miles is:
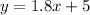
When x=0
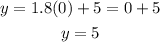
When x=10
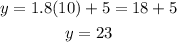
When x=20
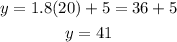
When x=30
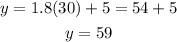
When x=40
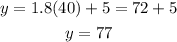
We can then fill the table using these values.
Part B
The y-intercept is the point at which x=0
From the table above, when x=0, y=5
• Therefore, the y-intercept is 5.
To use the table to find the slope, first, pick any two pair of points.
• For an example, I pick the points (0,5) and (10,23).
Then find the slope using the formula below: