Given:
![g(x)=(1)/(4)\sqrt[3]{x-3}+2](https://img.qammunity.org/2023/formulas/mathematics/college/ar7rljsqz56t7ygw9gso2du1snw2h7uw5v.png)
The function represents the number of users in millions who logged into a website since midnight.
1a. How many users have logged in by 9 am?
at 9am x = 9
Substitute x = 9 into g(x)
This gives
![g(9)=(1)/(4)\sqrt[3]{9-3}+2](https://img.qammunity.org/2023/formulas/mathematics/college/uldgbjxikgkvxpslcdwgtrxd5tp3gx2gis.png)
Simplify the expression
![\begin{gathered} g(9)=(1)/(4)\sqrt[3]{3}+2_{} \\ g(9)=(1)/(4)*1.44+2 \\ g(9)=2.36\text{ million} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/symt3tyn9sc99r1bfptbvgoyy0p3nirqut.png)
Therefore, 2360000 users have logged into the website by 9 am.
1b Domain and range of the function.
The domain of the function is the set of all input values for which the function is real and defined.
The values of x in g(x) starts from midnight
Hence at midnight x = 0, there are 24 hours in a day
Hence the domain of g(x) is
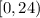
Range
The range of the function is the set of values of the dependent variables for which a function is defined
Since the function g(x) is defined for all dependent variables x
Then the range of the function is
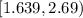
The range is in millions