Question

If the number of bacteria in a colony doubles every 237 hours and there is currently a population of 479,000 bacteria, what will the population be 948 hours from now?

Answer 1

Since the number of bacteria in a colony doubles every 237 hours, this growth has an exponential behavior. Every cicle of 237 hours, the population multiplies by 2. The following function models this behavior

`N(t)=N_0(2)^(t/237)`

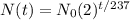
N_0 represents the initial value of the population and t represents the time in hours. There is currently a population of 479,000 bacteria, which means that our function is

`N(t)=479000(2)^(t/237)`

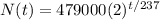
To find the population after 948, we just need to evaluate t = 948 in our function.

`\begin{gathered} N(948)=479000(2)^(948/237) \\ =479000(2)^4 \\ =479000\cdot16 \\ =7664000 \end{gathered}`

After 948, the population will be 7,664,000 bacteria.