Question

The larger of two numbers is 15 more than three times the smaller number. If the sum of the two numbers is 63, find the larger of the two numbers.

Answer 1

Information given

The larger of two numbers is 15 more than three times the smaller number. If the sum of the two numbers is 63, find the larger of the two numbers.​

Solution

Let x and y the two numbers and let's assume that y is the larger number so we have the following relation:

`y=15+3x`

the sum of th two numbers is :

`x+y=63`

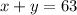
If we replace the first equation into the second one we got:

`x+15+3x=63`

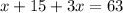
And solving for x we got:

`4x=48`
`x=(48)/(4)=12`

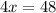
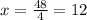
And the value of y would be:

`y=63-12=51`