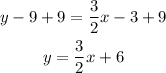Question

Question number 4Write the equation of a line passing through the given point and parallel to the given equation.Write your answer in slope-intercept form.(2,9)y=3/2x+3

Answer 1

You have to determine the equation of a line parallel to y=3/2x+3 that passes through the point (2,9)

The first step is to determine the slope of the line we have to determine.

Parallel lines have the same slope, the slope of the given line is m=3/2

Using the point-slope form you can determine the equation of the line as follows:

`y-y_1=m(x-x_1)`
`y-9=(3)/(2)(x-2)`

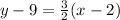
Now what's left is to write the equation in slope-intercept form.

Distribute the multiplication on the parentheses term

`\begin{gathered} y-9=(3)/(2)x-2\cdot(3)/(2) \\ y-9=(3)/(2)x-3 \end{gathered}`

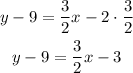
And pass 9 to the right side

`\begin{gathered} y-9+9=(3)/(2)x-3+9 \\ y=(3)/(2)x+6 \end{gathered}`