The standardized normal distribution has the following shape:
The horizontal axis is the z-value. The graph opens in both left and right ends to minus infinity and infinity, respectively, and the area under the whole curve is 1. Areas under the graph represent the probability that z takes certain values. (between two specific values, higher tan some value, or lower than some value).
Normal distribution tables allow us to calculate the area under the curve at the left of certain values of z. We are asked to find a value of z such that the area at its right is 0.08:
Usually, tables give values of z at the left of some value, so we need to rewrite the question. The total area under the curve is 1, and the area at the right of z is 0.08. This means that the area at the left of z is:
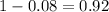
Then, we can say that we need to find the value of z that has an area at is left of 0.92.
If we read from a table of z-values, we can find that the value with an area of 0.92 at its left is approximately 1.4.