Question

Which of the following tables shows the correct steps to transform x^2 + 6x + 8 = 0 into the form (x − p)^2 = q?[p and q are integers]

Answer 1

`\begin{gathered} \text{Given} \\ x^2+6x+8=0 \end{gathered}`

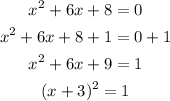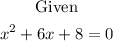
Determine the third term so that it is the perfect square of the trinomial.

Divide the coefficient of the second term, and square it. We get the following:

`\begin{gathered} 6x\Rightarrow6 \\ \\ \Big((6)/(2)\Big)^2 \\ =(3)^2 \\ =9 \end{gathered}`

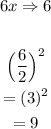
This means that the third term should be 9. Since we already have 8 as the third term of the given equation, add 1 on both sides to get the perfect square on the left side. Therefore, we have the following steps.

`\begin{gathered} x^2+6x+8=0 \\ x^2+6x+8+1=0+1 \\ x^2+6x+9=1 \\ (x+3)^2=1 \end{gathered}`