Question

Which lengths make sense for possible values of b

Answer 1

The first condition is for the perimeter of the equation so:

`P=2a+b=15.7`

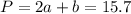
Now the secon condition is that the sum of two sides should be:

`\begin{gathered} a+b>b \\ b+b>a \end{gathered}`

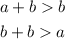
so the negative option and 0 don't make sence dor a distance, then we try with the value of b=0.5

So in the first equation will be:

`\begin{gathered} 2a+0.5=15.7 \\ 2a=15.2 \\ a=7.6 \end{gathered}`

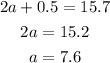
So the condition:

`\begin{gathered} b+b>a \\ 0.5+0.5>7.6 \end{gathered}`

this is false, so the solution is