Various possible side lengths of a right triangle are given.
It is required to choose which three will truly form a right triangle.
Recall the Pythagorean Theorem:
The Pythagorean Theorem states that in a right triangle, the relationship between the lengths of legs a and b and the length of the hypotenuse c is:

Check any three side lengths given. Notice that:
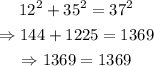
This implies that the three side lengths, 12, 35, and 37 satisfy the Pythagorean triple.
Hence, the three side lengths that can form a right triangle are 12 units, 35 units, and 37 units.
Select 12 units, 35 units, and 37 units.