The triangle formed by the system in the question is shown below:
The length of the shadow is represented by x.
We can use the Tangent Trigonometric ratio to solve for x.
The ratio is given as
From the diagram above, we have the following parameters:

Hence, we can substitute as

Solving for x, we have
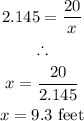
The correct answer is the FIRST OPTION (9.3 feet).