A bag has red, black and white stones.
There are 2 red stones.
Twice as many black as red, that means 4 black stones.
The remaining two-fifths of the stones are white.
That is,
If three-fifth of the stones are 6.
Then, two fifth of the stones are,
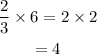
Therefore, there are 4 white stones.
The probability that the first stone is red and the second stone is white is,
Using the formula,
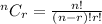
Here, n is the total number of items and r is the number of selected items.
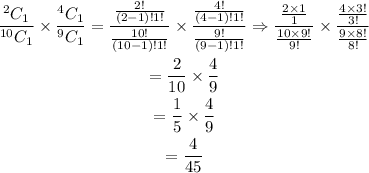
Hence, the correct option is B.
The probability that the first stone is red and the second stone is white is,
