Given the equation of the circle:

Let's draw a graph of the circle.
Apply the general equation of a circle:

Where:
(h, k) is the center of the circle.
r is the radius of the circle.
Rewrite 4 = 2²
Now, we have the equation:
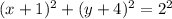
Thus, we have the following:
Center of the circle: (h, k) ==> (-1, -4)
Radius of the circle = 2.
Since the radius is 2, let's find the four points around the circumference of the circle.
We have:
• Point by the right: (-1 +2, -4) ==> (1, -4)
,
• Point by the left: (-1 -2, -4) ==> (-3, -4)
,
• Point at the top: (-1, -4 + 2) ==> (-1, -2)
,
• Point at the bottom: (-1, -4 - 2) ==> (-1, -6)
Therefore, the graph of the circle will be:
ANSWER: