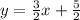Question

which equation represents a line on a coordinate plane that passes though the point (-1,1) and is parallel to the line 3x-2y=16?

Answer 1

Given equation of the parallel line:

3x - 2y = 16

The line passes through the point: (-1, 1)

Parallel lines have the same slope.

Let's rewrite the equation of the parallel line in slope intercetpt form:

y = mx + b

Where m is the slope and b is the y-intercept.

We have:

`\begin{gathered} 3x-2y=16 \\ \\ \text{Subtract 3x from both sides:} \\ -3x+3x-2y=-3x+16 \\ \\ -2y=-3x+16 \\ \\ \text{Divide all terms by -2:} \\ (-2y)/(-2)=(-3x)/(-2)+(16)/(2) \\ \\ y=(3)/(2)x+8 \end{gathered}`

The slope is 3/2

Substitute 3/2 for m in the slope intercept form.

`y=(3)/(2)x+b`

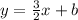
To solve for the y-intercept, b, since the line passes through (-1, 1), substitute -1 for x and 1 for y:

`\begin{gathered} y=(3)/(2)x+b \\ \\ 1=(3)/(2)(-1)+b \\ \\ 1=-(3)/(2)+b \\ \\ \text{Multiply all terms by 2:} \\ 1(2)=-(3)/(2)\ast2+2(b) \\ \\ 2=-3+2b \\ \\ \text{Add 3 to both sides:} \\ 3+2=-3+3+2b \\ \\ 5=2b \\ \\ \text{Divide both sides by 2:} \\ (5)/(2)=(2b)/(2) \\ \\ (5)/(2)=b \\ \\ b=(5)/(2) \end{gathered}`

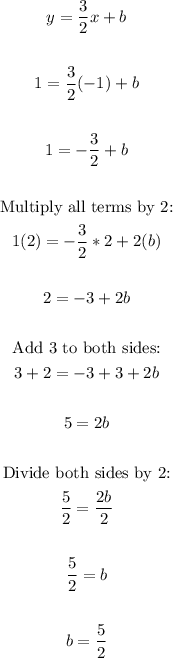
The y-intercept, b is 5/2

Therefore, the equation that represents the line is:

`y=(3)/(2)x+(5)/(2)`

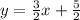
ANSWER:

`y=(3)/(2)x+(5)/(2)`