Given:
Area of the triangle, A = 70 square meters
Base of the triangle, b = 10 meters
To find the height, h, of the triangle, use the area of the triangle formula below:
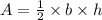
Rewrite the formula for h:
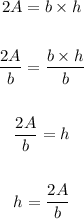
To find the height, h, we have the equation:
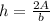
Input values into the equation:

Therefore, the height of the triangle is 14 meters
ANSWER:
14 meters