Question

Graph the parametre ve including an arrow to show the direction of the curveyo

Answer 1

We have the following function (defined in terms of two parametric equations):

`\begin{gathered} x(t)=-t \\ y(t)=\sqrt[]{t} \end{gathered}`

In order to know which function we have to select from the options we can do the following trick.

1) We compute the square of the second function:

`y^2=(\sqrt[]{t})^2=t`

2) We write the first function in terms of the last equation:

`x=-t=-(y^2)=-y^2`

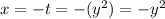
3) We proceed to analize the last equation:

- We see that the graph must have negatives values of x for any value of y. So the correct graphs must be the ones that respect this. So the answer must be option 1 or 2.

- We also see from the equation of y that t must be positive, so the range of values of t is:

`0\leq t`

If we increase the value of t from 0 to any value that we want, the value of y increases and because of the relation of x and y, the value of x must be more negative for higher values of t or y. So the correct option is the one with the arrow to the left, option 1.