Question

The premiter of a rectangle Is 86 inches. If it's length is 2 inches shorter than it's width, find the dimensions

Answer 1

The length of rectangle be L and width be W. So the relation length and width is,

`L=W-2`

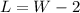
The perimeter is 86, so equation is

`P=2(L+W)`

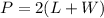
Substitute the values in the equation to obtain the value of width.

`\begin{gathered} 86=2(W-2+W) \\ 2W-2=43 \\ W=(45)/(2) \end{gathered}`

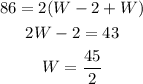
Susbtitute the value of width in equation to obtain the length of rectangle.

`\begin{gathered} L=(45)/(2)-2 \\ =(45-4)/(2) \\ =(41)/(2) \end{gathered}`

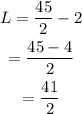
So length of rectangle is 41/2 and width is 41/2.