Question

given the function g(x) = -x^2 + 5x + 14 determine the average rate of change of the function over the interval 1 ≤ x ≤ 9

Answer 1

Given:

Given the function is as

`g\mleft(x\mright)=-x^2+5x+14=y`

Required:

We want to determine the average rate of change of the function over the interval 1 ≤ x ≤ 9

Step-by-step explanation:

In general the rate of change of given function is

`(dy)/(dx)=-2x+5`

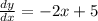
Now at x=1

`(dy)/(dx)=-2+5=3`

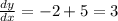
and at x=9

`(dy)/(dx)=-18+5=-13`

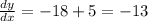
between x=1 and x=9, dy/dx changes by

`-13-3=-16`

and distance between x=1 and x=9 is

`9-1=8`

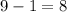
average rate change is

`-(16)/(8)=-2`

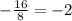
FInal answer:

-2