ANSWER
4 units
Step-by-step explanation
The transformations made to the line segment XY are a reflection and a translation. Both of these transformations do not change the size of the figure, so the length of line segment X'Y' is the same as the length of line segment XY.
The distance between two points (x₁, y₁) and (x₂, y₂) is found with the Pythagorean Theorem,
![d=√((x_1-x_2)^2+(y_1-y_2)^^2)]()
In this case, the endpoints of line segment XY are (-6, 4) and (-2, 4), so its length is,
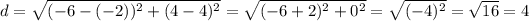
Hence, the length of line segment X'Y' is 4 units.