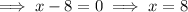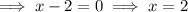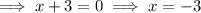If f(x)=x^3-7x^2-14x+48 and f(8)=0, then find all of the zeros of f(x) algebraically

Question

asked Nov 25, 2023 211k views

1 Answer

Fresh · Answer 1 · 2023-12-01T03:27:30+0000

Answer:

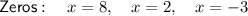
$\textsf{Zeros}: \quad x=8, \quad x=2, \quad x=-3$

Explanation:

Given function:

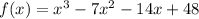
f(x)=x^3-7x^2-14x+48

Factor Theorem

If f(x) is a polynomial, and f(a) = 0, then (x – a) is a factor of f(x).

Therefore, since f(8) = 0, then (x - 8) is a factor of the function f(x):

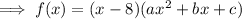
$\implies f(x)=(x-8)(ax^2+bx+c)$

The coefficient of x³ is a.

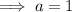
$\implies a=1$

The constant 48 is equal to -8c.

$\implies c=-6$

To find the value of b:

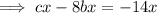
$\implies cx - 8bx = -14x$

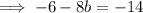
$\implies -6-8b=-14$

$\implies -8b=-8$

$\implies b=1$

Therefore:

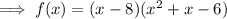
$\implies f(x)=(x-8)(x^2+x-6)$

Factor (x² + x - 6):

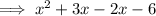
$\implies x^2+3x-2x-6$

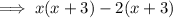
$\implies x(x+3)-2(x+3)$

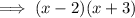
$\implies (x-2)(x+3)$

Therefore the fully factored function is:

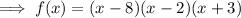
$\implies f(x)=(x-8)(x-2)(x+3)$

To find the zeros of the function, set each factor to zero:

$\implies x-8=0 \implies x=8$

$\implies x-2=0 \implies x=2$

$\implies x+3=0 \implies x=-3$