Question

Below are the times (in days) it takes for a sample of 5 customers from Tony's computer store to pay their invoices.

Answer 1

In this problem, we have the following data sample:

`32,37,24,22,20.`

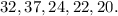
We must compute the standard deviation of this data sample.

1) First, we compute the mean value which is given by the following formula:

`\bar{x}=(\sum^n_(i\mathop=1)x_i)/(n)=(32+37+24+22+20)/(5)=(135)/(5)=27.`

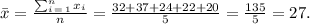
2) Now, we compute the standard deviation using the following formula:

`\sigma=\sqrt[]{\frac{\sum^n_{i\mathop{=}1}(x_i-\bar{x})^2}{n-1}}=\sqrt[]{(208)/(5-1)}\cong7.21.`

Answer

The standard deviation is 7.21.