Answer:
The nature of the result of the given expression is irrational. (Option 2)
Step-by-step explanation:
Let's simplify the given expression by rationalizing the denominator. To rationalize the denominator, multiply both numerator and denominator by √2.
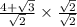
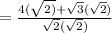
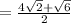
The result is shown above. When converted to a decimal number, it is equivalent to 4.053171996.
As we can see, the result is neither an integer nor a whole number because these two don't have decimal points.
In addition, the result is not a rational number because the equivalent decimal number is both non-terminating and non-repeating decimal.
Hence, the result is an irrational number. (Option 2)