We have to graph the function:
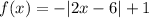
To do that we can start by looking at which point the absolute function changes direction.
This happens when 2x-6=0.
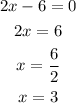
We have one line for x<3 and other line, with different slope, for x>3.
Then we define the function as a piecewise function:
For x<3, we have:

And for x>3:
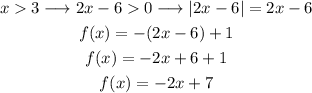
We can resume the function f(x) as:
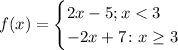
For x<3, we have a positive-slope line (m=2), with y-intercept b=-5.
For x>3, we have a negative-slope line (m=-2), with y-intercept b=7 (but as x>3, the y-axis is not intercepted by this line). We also can use the reference that, when x=3, y=1.
We can graph this as:
NOTE: I draw them with different colours for each part of the function, but it is the same function and should be drawn with the same colour.