Question

TGA graph shows y in direct variation with x. Find the value of y when x = -15.Option D. -12

Answer 1

Given the graph of the Direct Variation, you can identify this point:

`\mleft(2,4\mright)`

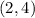
By definition, the equation of a Direct Variation has this form:

`y=kx`

Where "k" is the Constant of Variation.

In this case, knowing the point shown before, you can set up that:

`\begin{gathered} x=2 \\ y=4 \end{gathered}`

Now you can substitute these values into the equation and solve for "k":

`\begin{gathered} 4=k(2) \\ \\ (4)/(2)=k \\ \\ k=2 \end{gathered}`

Therefore, the equation that represents the line given in the exercise is:

`y=2x`

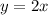
Since you need to find the value of "y" when:

`x=-15`

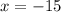
You need to substitute that x-value into the equation and evaluate:

`\begin{gathered} y=2(-15) \\ y=-30 \end{gathered}`

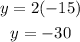
Hence, the answer is: Option A.