Given:
Percentage of people over 65 = 53%
Sample = 15
Let's solve for the following:
• (a). Exactly 8 of the patients were over the age of 65 when they had their surgery?
Here, we are to apply binomial probability.
We have:
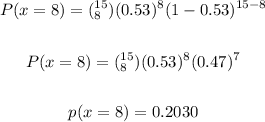
The probability is 0.2030
• (b). Fewer than 10 of the patients were over the age of 65 when they had their surgery?
Here, we are to find P(x < 10).
Using binomial probability, we have:
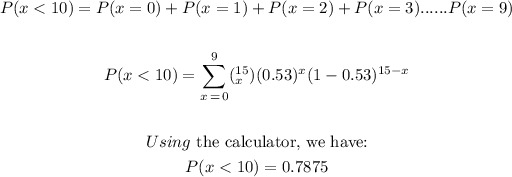
The probability is 0.7875
• (c). Between 6 and 11 of the patients were over the age of 65 when they had their surgery?
Since between does not include the endpoints of the interval, we are to find:
P(6 < x < 11) = P(x = 7) + P(x = 8) + P(x = 9) + P(x = 10)
Hence, we have:
![\begin{gathered} P(6<strong>The probability is </strong><strong> 0.6814 </strong><p>• (d). More than 12 of the patients were over the age of 65 when they had their surgery?</p><p>We are to find P(x > 12) = P(x = 13) + P(x = 14) + p(x = 15)</p>[tex]\begin{gathered} P(x>12)=\sum_{x\mathop{=}13}^(12)(_x^(15))(0.53)^x(1-0.53)^(15-x) \\ \\ P(x>12)=0.0071 \end{gathered}]()
• (e). Would it be unusual that all 15 of the patients were over the age of 65 when they had their surgery?
We are to find P(x = 15):
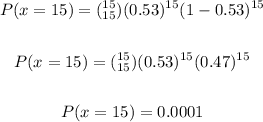
ANSWER:
• (a). 0.2030
,
• (b). 0.7875
,
• (c). 0.6814
,
• (d). 0.0071
,
• (e). 0.0001