ANSWER:
A. no solution
Step-by-step explanation:
Given:
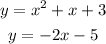
To determine the solutions of the given system of equations, we'll equation both equations as seen below;
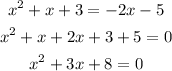
Recall that a quadratic equation in standard form is generally given as;

If we compare both equations, we can see that a = 1, b = 3 and c = 8
We can use the below discriminant of a quadratic equation to determine the number of solutions as seen below;

We can see that the determinant is less than zero and this means that the equation will have no real solutions, therefore we can say that the system of equations has no solutions