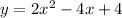Question

Write the standard form equation for the function that has a vertex: (1, 2); passes through (3, 10).Write the equation of the parabola.I worked out the problem and for some reason I’m getting the same answer and it’s wrong. I don’t know why

Answer 1

Given:

The vertex of the parabola is (1,2).

The point passes through the parabola = (3,10).

Required:

We need to find the equation of the parabola.

Step-by-step explanation:

Consider the standard form equation for the parabola.

`y=a(x-h)^2+k`

where (h,k) is the vertex.

Substitute (h,k) =(1,2) in the equation.

`y=a(x-1)^2+2`

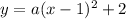
Substitute x =3 and y=10 in the equation to find the value of a.

`10=a(3-1)^2+2`

`10=a(2)^2+2`

`10=4a+2`

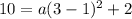
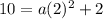
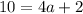
Subtract 2 from both sides of the equation.

`10-2=4a+2-2`

`a=2`

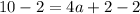
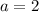
Substitute a =2, h=1 nad l=2 in the equation of the parabola.

`y=2(x-1)^2+2`
`\text{Use \lparen a-b\rparen}^2=a^2+b^2-2ab.`

`y=2(x^2+1^2-2(1)(x))+2`

`y=2(x^2+1-2x)+2`

`y=2x^2+2*1-2*2x+2`

`y=2x^2+2-4x+2`

`y=2x^2-4x+2+2`

`y=2x^2-4x+4`

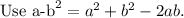
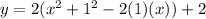
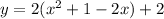
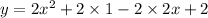
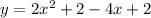
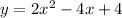
Final answer:

The standard form equation for the function:

`y=2x^2-4x+4`