Question

Two cars are headed in the same direction on the HWY. The trailing car is moving at14.6 m/s and has a mass of 1,230 kg. The lead car is moving at 13 m/s and has a massof 1,278 kg. The trailing car runs into the lead car and bumps it. Afterwards, the trailingcar has a velocity of 13.6 m/s.To determine if the collision is elastic, calculateΔKE = ΚΕ' – KE

Answer 1

The initial momentum of the system is,

`p_i=m_1u_1+m_2u_2`

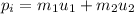
The final momentum of the system is,

`p_f=m_1v_1+m_2v_2`

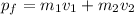
According to conservation of momentum,

`p_i=p_f`

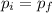
Plug in the known values,

`m_1u_{1_{}}+m_2u_2=m_1v_1+m_2v_2`

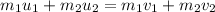
Substitute the known values,

`\begin{gathered} (1230kg)(14.6m/s)+(1278kg)(13m/s)=(1230kg)(13.6m/s)+(1278kg)v_2 \\ 17958\text{ kgm/s+}16614\text{ kgm/s=}16728\text{ kgm/s+}(1278kg)v_2 \\ v_2=\frac{34572\text{ kgm/s-}16728\text{ kgm/s}}{1278\text{ kg}} \\ =13.96\text{ m/s} \end{gathered}`

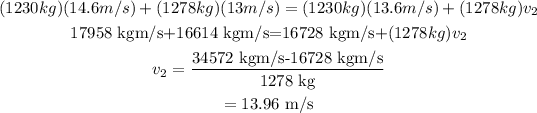
The initial kinetic energy of the system is,

`K=(1)/(2)m_1u^2_1+(1)/(2)m_2u^{2^{}}_{2^{}}`

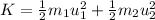
The final kinetic energy of the system is,

`K^(\prime)=(1)/(2)m_1v^2_1+(1)/(2)m_2v^{2^{}}_{2^{}}`

The change in kinetic energy of the system is,

`\Delta K=K^(\prime)-K`

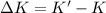
Plug in the known expressions,

`\begin{gathered} \Delta K=(1)/(2)m_2v^2_2+(1)/(2)m_1v^2_1-(1)/(2)m_2u^2_2-(1)/(2)m_1u^2_1 \\ =(1)/(2)m_2(v^2_2-v^2_1)+(1)/(2)m_1(v^2_1-u^2_1) \end{gathered}`

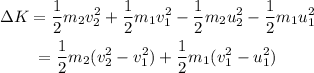
Substitute the known values,

`\begin{gathered} \Delta K=(1)/(2)(1278kg)((13.96m/s)^2-(13m/s)^2)+ \\ (1)/(2)(1230kg)((13.6m/s)^2-(14.6m/s)^2) \\ =(639\text{ kg)(}194.88m^2s^(-2)-169m^2s^(-2))+(615\text{ kg)(}184.96m^2s^(-2)-213.16m^2s^(-2)) \\ =(639\text{ kg)(}25.88\text{ }m^2s^(-2))(\frac{1\text{ J}}{1\text{ kg}m^2s^(-2)})+(615\text{ kg)(}-28.2m^2s^(-2))(\frac{1\text{ J}}{1\text{ kg}m^2s^(-2)}) \\ =16537.32\text{ J-}17343\text{ J} \\ =-805.68\text{ J} \end{gathered}`

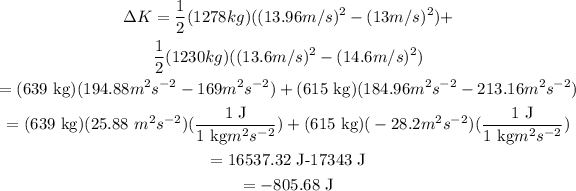
Therefore, the change in kinetic energy of the system is -805.68 J.