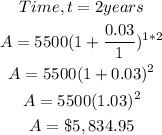Question

Suppose that a loan of $5500 is given at an interest rate of 3% compounded each year.Assume that no payments are made on the loan. Follow the instructions below do not do any rounding. A/ find the amount owed at the end of one year B/ find the amount owed at the end of two years

Answer 1

A) $5,665

B) $5,834.95

Step-by-step explanation:

We were given that:

Loan, P = $5,500

Interest Rate, r = 3% = 3/100 = 0.03

Compounding, n = annually = 1

Time, t = ?

The amount owed is obtained using the formula:

`A=P(1+(r)/(n))^(nt)`

A) At the end of one year:

`\begin{gathered} Time,t=1year \\ A=5500(1+(0.03)/(1))^(1*1) \\ A=5500(1+0.03) \\ A=5500(1.03) \\ A=\text{\$}5,665 \end{gathered}`

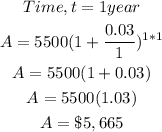
B) At the end of two years:

`\begin{gathered} Time,t=2years \\ A=5500(1+(0.03)/(1))^(1*2) \\ A=5500(1+0.03)^2 \\ A=5500(1.03)^2 \\ A=\text{\$}5,834.95 \end{gathered}`