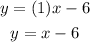Answer:
y = x - 6
Step-by-step explanation:
The slope-intercept form of the equation of a line is generally given as;

where m = the slope of the line
b = y-intercept of the line
Given the slope(m) as 1 and the point (7, 1) where x = 7 and y = 1, we'll substitute these values into our slope-intercept equation and solve for b as seen below;

Since we have m = 1 and b = -6, the equation of the given line in slope-intercept form can now be written as;