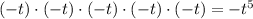Remember that when two negative numbers are multiplied, the result is positive, while if a negative number is multiplied by a positive number, the result is negative.
If an expression has an even amount of negative factors, then the product is positive. If an expression has an odd amount of negative factors, then the product is negative.
In the given expression:
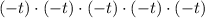
There are five negative factors. Then, the result must be negative. Recalling the properties of exponentials, we can rewrite this expression as:
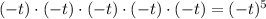
Which is equal to:
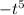
Therefore: