Answer:
The object will hit the ground 5 seconds after it is thrown.
Explanation:
Given equation:
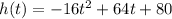
where:
- h is the height of the object from the ground (in feet).
- t is time (in seconds).
The object will hit the ground when the height of the object is zero.
Therefore, to find the time when the object hits to ground, set the equation to zero and solve for t.
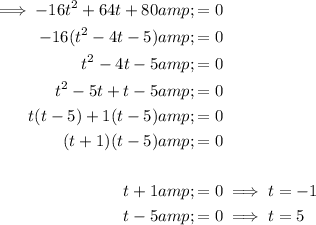
As t ≥ 0, t = 5 only.
Therefore, the object will hit the ground 5 seconds after it is thrown.