1) To build a 30-60-90 triangle, we start with an equilateral triangle with sides of length 4:
2) Now, we split the triangle with a vertical line:
3) From trigonometry, we know that:
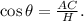
Where:
• θ is the angle of interest,
,
• AC is the adjacent cathetus to the angle θ,
,
• H is the hypotenuse.
In this case, we have:
• θ = 60°,
,
• AC = 2,
,
• H = 4.
Replacing these data in the formula above, we get:
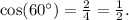
Answer: 1/2