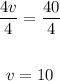Given the following equation:
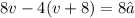
The steps to solve for "v" are the shown below:
1.Apply the Distributive property:
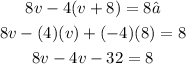
2. Apply the Addition property of equality by adding 32 to both sides of the equation:

3. Add the like terms:
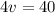
4. Apply the Division property of equality by dividing both sides of the equation by 4.
The solution is: