The general slope-intercept form of a linear equation is:

----------------------------------------
You have a linear equation parallel to:
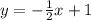
That means that both equations have the same slope
m= - 1/2
If the equation passes through (-4 , -1) we can use that point to find the value of the b in the slope-intercept form:
(-4 , -1) x= - 4 y= - 1
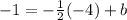
We clear the b:
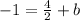
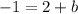
Then you know the values of
m=-1/2
b=-3
The equation in slope-intercept form is:

To graph a linear equation you need two point, as you have (-4, -1) and the point of the y-intercept that is (0,b) = (0,-3)
You put in the plane the two points:
And you draw a line that link the two points to get the final graph: