SOLUTION
Step 1 :
We need to know how large a sample is necessary.
Let p denotes the United States Homes have a direct satellite TV Receiver is 25 % or 0. 25.
From the information, we know that E is 3 % or 0.03 and the confidence interval of 95%.
When p is known, then the sample size formula is :
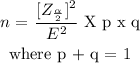
When p is unknown, then the sample size formula is:
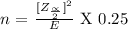
Now, we have to determine:
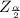
The confidence level is :
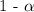
We know that:


If we use the known p, then the sample size is :

If we use the known p, then the sample size is 801.
Therefore, a sample n is 801.
Step 2 :
If we use the un-known p , then the sample size is :
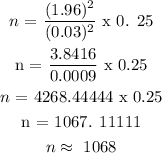
If we use the unknown p, then the sample size is approximately 1068.
Therefore, a sample n is 1068.