Given:
The objective is to find the area of triangle ABC and XYZ.
Step-by-step explanation:
The general formula to find the area of a triangle is,

To find the area of triangle ABC:
The height of the triangle DC can be calculated using the Pythagorean theorem of triangle ADC.
![DC=\sqrt[]{AC^2-AD^2}\ldots.\text{ .(2)}](https://img.qammunity.org/2023/formulas/mathematics/high-school/gav6af3ybgobu6tn1dmqb33s9nd2rdrkl1.png)
On plugging the given values in equation (2),
![\begin{gathered} D\C=\sqrt[]{13^3-5^2} \\ =\sqrt[]{169-25} \\ =\sqrt[]{144} \\ =12 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/high-school/z1oeqjaeqduii0y953ojsavkj8wzuxjrzd.png)
Thus, the height of triangle ABC is 12.
Since it is given in the figure that AD = DB = 5.
So the base of the triangle AB = 5 + 5 = 10.
Now, substitute the obtained values in equation (1).
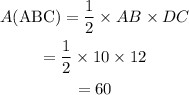
To find the area of triangle XYZ:
Since it is given in the figure that XW= WY = 15.
So the base of the triangle XY = 15 + 15 = 30.
The height of the triangle is WZ = 36.
Now, substitute the obtained values in equation (1).
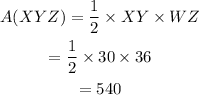
Hence, the area of triangle ABC is 60 square units and the area of triangle XYZ is 540 square units.