Question

Instructions: Match the following with the best method to use to solve the given system of linear equations.

Answer 1

Given:

System of linear equations.

Step-by-step explanation:

System 1:

`\begin{gathered} y\text{ = 3x + 15 } \\ y\text{ = 2x + 11} \end{gathered}`

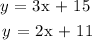
The above system of equations can be simplified ELIMINATION BY SUBTRACTION.

System 2:

`\begin{gathered} 7x\text{ - 2y = 0} \\ y\text{ = 6x + 5} \end{gathered}`

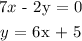
The above system of equations can be simplified using the SUBSTITUTION METHOD.

System 3:

`\begin{gathered} -3x\text{ - 5y = -5} \\ -3x\text{ + 5y = 5} \end{gathered}`

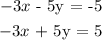
The above system of the equation can be simplified using ELIMINATION BY SUBTRACTION.

System 4:

`\begin{gathered} -5x\text{ - 4y = -6} \\ -7x\text{ - 4y = -2} \end{gathered}`

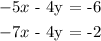
The above system of the equation can be simplified using ELIMINATION BY SUBTRACTION.

System 5:

`\begin{gathered} 5x\text{ + 2y = 0} \\ 15x\text{ + 7y = 0} \end{gathered}`

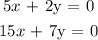
The above system of the equation can be simplified using ELIMINATION BY MULTIPLICATION.