Question

Graph the equation after rewriting it on in slope intercept form. 2y-4x=3

Answer 1

`\begin{gathered} \text{Given} \\ 2y-4x=3 \end{gathered}`

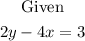
The slope intercept form is defined as

`\begin{gathered} y=mx+b \\ \text{where} \\ m\text{ is the slope} \\ b\text{ is the y-intercept} \end{gathered}`

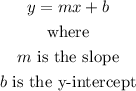
Rearrange the given equation by transferring the x term to the left side and dividing both sides by 2.

`\begin{gathered} 2y-4x=3 \\ 2y=4x+3 \\ (2y)/(2)=(4x+3)/(2) \\ y=2x+(3)/(2) \\ \\ \text{Therefore, the slope intercept form of the equation }2y-4x=3\text{ is} \\ y=2x+(3)/(2) \end{gathered}`

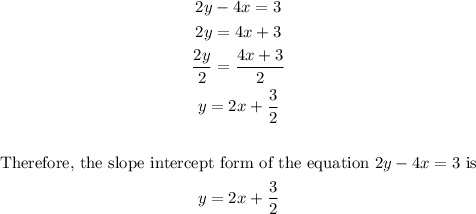
Now that we have the slope intercept form, get at least any of the two points of the equation by substituting any two values of x, for this case, we will use x = 0, and x = 1

`\begin{gathered} \text{If }x=0 \\ y=2x+(3)/(2) \\ y=2(0)+(3)/(2) \\ y=(3)/(2) \\ y=1.5\rightarrow(0,1.5)\rightarrow\text{first point} \\ \\ \text{If }x=1 \\ y=2x+(3)/(2) \\ y=2(1)+(3)/(2) \\ y=2+(3)/(2) \\ y=(7)/(2) \\ y=3.5\rightarrow(1,3.5)\rightarrow\text{second point} \end{gathered}`

Now that we have two coordinates (0, 1.5) and (1, 3.5), plot the two points in the graph, and then connect a line between them. This will result with the graph.