When simplifying power of a power expression, simply multiply the two powers.
Let's start with the first expression.
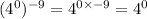
Hence, the first expression matched with 4⁰.
Moving on to the second expression,
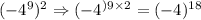
Hence, the second expression match with (-4)¹⁸.
For the third expression,
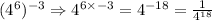
Hence, the third expression match with 1/4¹⁸.
Lastly, moving on to the 4th expression,
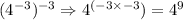
Hence, the 4th expression match with 4⁹.