Question

Jackson has a coin collection consisting of quarters and dimes. The total value of his collection is $13.75. His collection is consists of five less quarters than two times the number of dimes. Using the variables q and d to represent the number quarter in his collection and the number of dime in his collection respectively, determine a system of equations that describe the situation.(a)Enter the equations below separated by comma (b)How many dimes are in his collection ?(c)How many quarters are in his collection?

Answer 1

• (a)0.25q+0.1d=13.75, q=2d-5

,

• (b)d=25

,

• (c)q=45

Explanation:

Let the number of quarters in his collection = q

Let the number of dimes in his collection = d

• 1 quarter = $0.25

,

• 1 dime =$0.10

The total value of his collection = $13.75.

`0.25q+0.1d=13.75\cdots(1)`

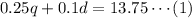
He has five fewer quarters than two times the number of dimes.

`q=2d-5\cdots(2)`

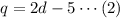
The system of equations that describes the situation is:

`\begin{gathered} 0.25q+0.1d=13.75 \\ q=2d-5 \end{gathered}`

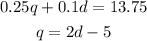
Next, we solve for q and d.

Substitute the second equation into the first.

Finally, solve for q.

`\begin{gathered} q=2d-5 \\ q=2(25)-5 \\ q=45 \end{gathered}`

Jackson has 25 dimes and 45 quarters in his collection.