Question

Write an equation of the line that passes through each pairs of points y=mx+b

Answer 1

Equation of a straight line can be represented as y = mx + c where m is slope and c is y-intercept

slope, m of the line is calculated as follows:

`\begin{gathered} slope,m\text{ = }\frac{\text{change in y}}{\text{change in x}} \\ \\ m\text{ = }(-4-2)/(-7-5) \\ m=(-6)/(-12) \\ m=(1)/(2) \end{gathered}`
`\begin{gathered} to\text{ calculate the equation of the line:} \\ m\text{ = }(1)/(2),\text{ a point on the line is (5, 2)} \\ x1=\text{ 5, y1 =2 } \\ \text{ using y-y1=m(x-x1)} \\ y-2=(1)/(2)(x-5) \\ y-2=(x)/(2)-(5)/(2) \\ y\text{ = }(x)/(2)-(5)/(2)\text{ +2} \\ y\text{ = }(x)/(2)-(1)/(2) \\ \text{The equation of the line is }y\text{ = }(x)/(2)-(1)/(2) \end{gathered}`

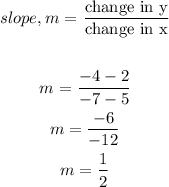
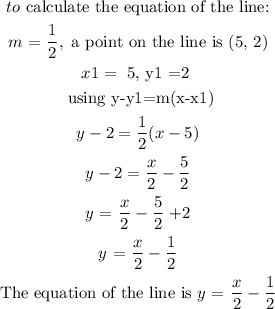
The slope is 1/2

For the y-intercept:

Compare the equation with y = mx + c

c = -1/2

The y-intercept is -1/2