Question

Part A: complete the statement below about inscribed quadrilateralsPart B: select all of the statements that are true

Answer 1

Because the quadrilateral is incribed in a circle, the opposite vetices forms supplementary arcs of the circle, which means that opposite angles of it are supplementary.

This means that the first option is opposite angles and the second is supplementary.

They being supplementaty means that their sum is equal to 180°, so:

`\begin{gathered} m\angle A+m\angle C=180\degree \\ m\angle B+m\angle D=180\degree \end{gathered}`

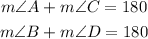
However, notrhing can be said about congruency of opposite angles, so the first option of part be is not necessarily true.

The second is true, as we showed above.

The third shows a relationship between adjacent angle, which is not necessarily true.

The fourth is false because the opposite angles should add to 180° no 90°.

The last is a property of all quadrilaterals (independetly of them being inscribed or not): the sum of its internal angles is equal to 360°, so the fifth option is true.

So, in part B the only true statements are the second and the fifth.