Question

Suppose that two objects attract each other with a gravitational force of 16 N. If the mass

of both objects was doubled, and if the distance between the objects remained the same,
then what would be the new force of attraction between the two objects?

Answer 1

F' = 64 N

Explanation:

The force of gravitation between two objects is given by Newton's Law of Gravitation as follows:

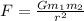`F = (Gm_(1)m_(2))/(r^2)\\`______________ equation (1)

where,

F = Force = 16 N

G = universal gravitational constant

m₁ = mass of the first object

m₂ = mass of the second object

r = distance between objects

Now, the masses of each object are doubled and the distance between them is same:

m₁' = 2m₁

m₂' = 2m₂

r' = r

Hence, the new force will be:

`F' = (G(2m_(1))(2m_(2)))/(r^2)\\\\F' = 4(Gm_(1)m_(2))/(r^2)`

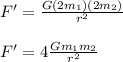
using equation (1), we get:

F' = 4 F = 4(16 N)

F' = 64 N