Given:
The equation is,
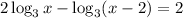
Step-by-step explanation:
Simplify the equation by using logarthimic property.
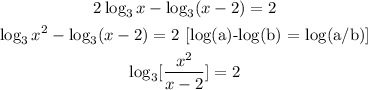
Simplify further.
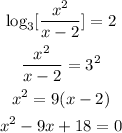
Solve the quadratic equation for x.
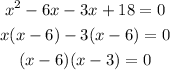
From the above equation (x - 6) = 0 or (x - 3) = 0.
For (x - 6) = 0,
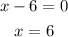
For (x - 3) = 0,
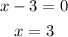
The values of x from solving the equations are x = 3 and x = 6.
Substitute the values of x in the equation to check answers are valid or not.
For x = 3,
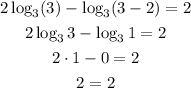
Equation satisfy for x = 3. So x = 3 is valid value of x.
For x = 6,
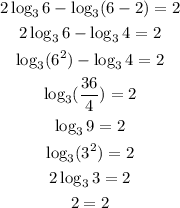
Equation satifies for x = 6.
Thus values of x for equation are x = 3 and x = 6.