From the problem, we have the vertices of the triangle :
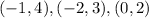
We need to find the distances between these vertices.
Perimeter is the sum of all sides of the triangle.
Using distance formula :
![d=\sqrt[]{(x_2-x_1)^2+(y_2-y_1)^2}](https://img.qammunity.org/2023/formulas/mathematics/college/be685jmxw05hm2tq94m5iuge2xjynn1hfn.png)
The distances are :
![\begin{gathered} d_1=\sqrt[]{(-2+1)^2+(3-4)^2}=\sqrt[]{2} \\ d_2=\sqrt[]{(0+1)^2+(2-4)^2}=\sqrt[]{5} \\ d_3=\sqrt[]{(0+2)^2+(2-3)^2}=\sqrt[]{5} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/g4u2g6stc13ddq5stdaxkxs94upui01rqb.png)
Take the sum of the distances :
![\begin{gathered} P=\sqrt[]{2}+\sqrt[]{5}+\sqrt[]{5} \\ P=\sqrt[]{2}+2\sqrt[]{5}\quad or\quad 5.89 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/divyjv9ka2tpcooncl9fq4xm35o6orr1ws.png)
ANSWER :
P = (√2 + 2√5) units or 5.89 units