The inequality function is given .
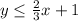
To draw the graph of the inequality function , first determine the coordinates of the function.
At x equal to 0.
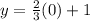
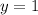
At y equal to 0.
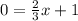

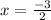

The coordinates at which the function satisfy are obtained as,
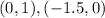
For the inequality of the function given is less than equal to that means the shaded region of the graph lies below the origin .
Therefore the garph of the inequality of the function is,
According to the options given A,B,C and D.
The correct option is A.
Reason : The ineqaulity graph is always represented by dashed line.