Answer:
Area = 640 sq. meters
Step-by-step explanation:
5.
We are given the figure of a prism and we are to obtain its surface area
The prism has 5 faces: 2 triangular shaped faces of equal dimensions, 3 rectangular faces of different dimensions (2 out of these 3 have the same dimension while the third has a different dimension)
For the triangular-shaped face(s), its/their dimension is:
Height = 12 inches
Base = 5 inches
For the rectangular-shaped faces, their dimensions are:
Rectangle 1 & 2: Length = 20 inches, Width = 12 inches
Rectangle 3: Length = 20 inches, Width = 5 inches
The surface area of the prism is obtained by the sum of all the areas of the 5 faces itemized above. This is shown below:

The area of the prism is obtained thus:
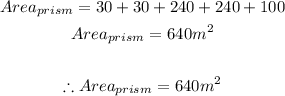
Therefore, the area of the prism is 640 square meters