Question

Bill forgets to put his car in park and it starts rolling west. When it is moving at a speed of 3.5m/s, it collides with Tanya’s car, which is moving at a speed of 2m/s east. Bill’s car bounces backward (to the east) at a speed of 2m/s. Bill’s car has a mass of 900kg, and Tanya’s car has a mass of 1100kg. A. What is the velocity of Tanya’s car after the collision?B. Assuming all lost kinetic energy is converted to hear during the collision?

Answer 1

For A.

Bill's car

mass=900 kg

speed=3.5m/s towards west

Tanya's car

mass = 1100 kg

speed = 2 m/s towards East

The momentum

`P=P1-P2`

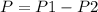
For P1 and P2

`P_1=900(3.5)=3150\text{ kg m/s}`

`P=3150-2200=950\text{ kgm/s}`

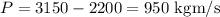
then for the velocity, we have the final momentum

We can calculte the final momentum of Bill's

`P=900(2)=1800\text{ kgm/s}`

`1100v-1800=950`

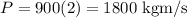
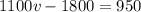
v is the velocity of Tanya’s car after the collision, so we need to isolate the v

`v=(950+1800)/(1100)=2.5\text{ m/s }`

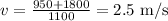
The velocity of Tanya's car after the collision is 2.5 m/s towards west

For B.

First, we need to calculate the kinetic energy before the collision of Bill's car and Tanya's car

`KE_B=(1)/(2)m_Bv^2_B+=(1)/(2)m_Tv_T`
`KE_B=(1)/(2)(900)(3.5)^2+(1)/(2)(1100)(2)^2=5512.5+2200=7712.5J`

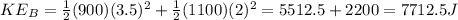
Then the kinetic energy after the collision

`KE=(1)/(2)(900)(2)^2+(1)/(2)(1100)(2.5)^2=1800+3437.5=5237.5`

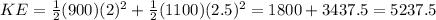
then

`7712.5-5237.5=2475\text{ J}`

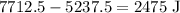
the energy that was converted to heat was 2475J